Using redundant ancilla encoding and flags for very low overhead fault-tolerant magic state preparation
2020
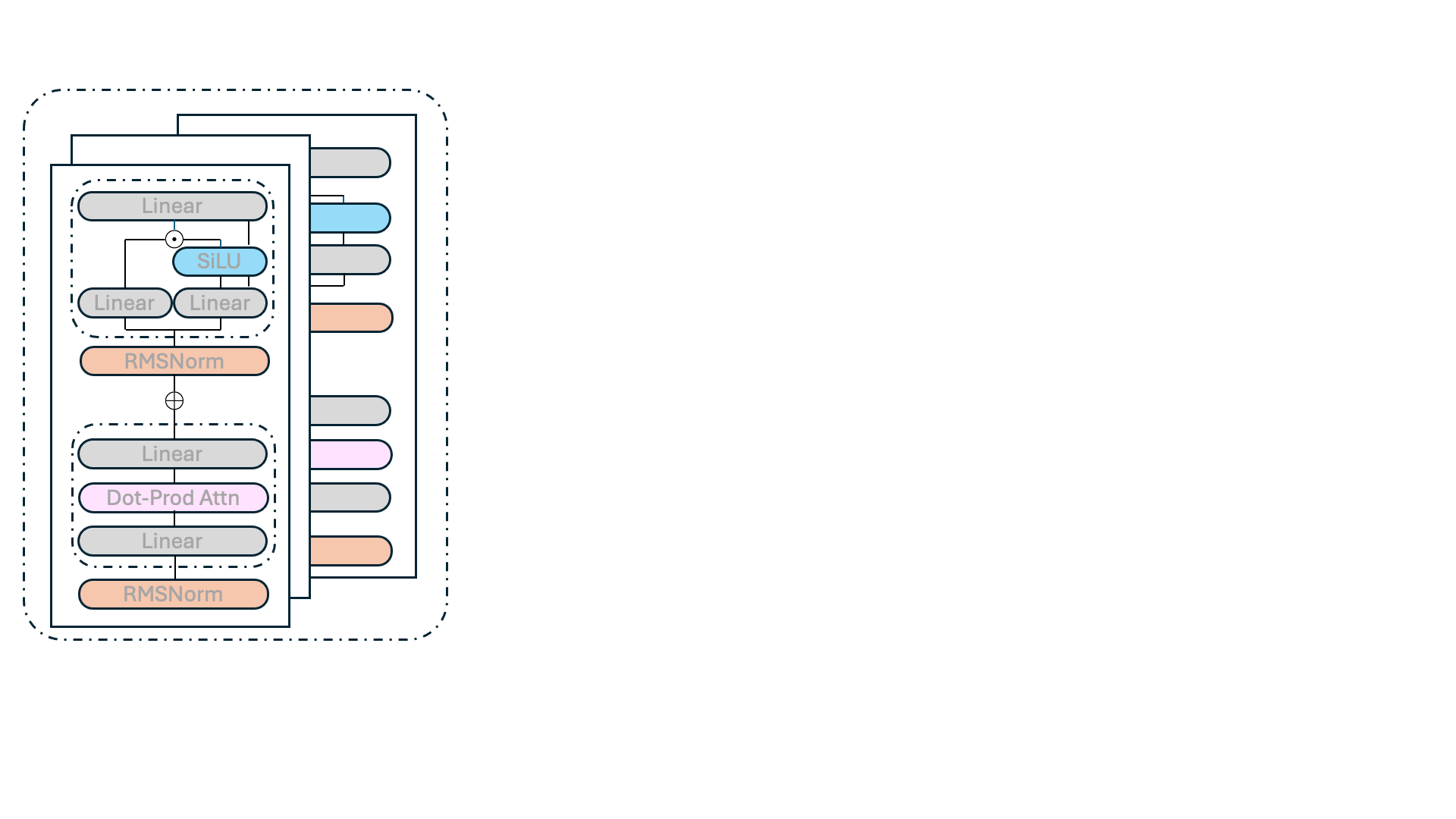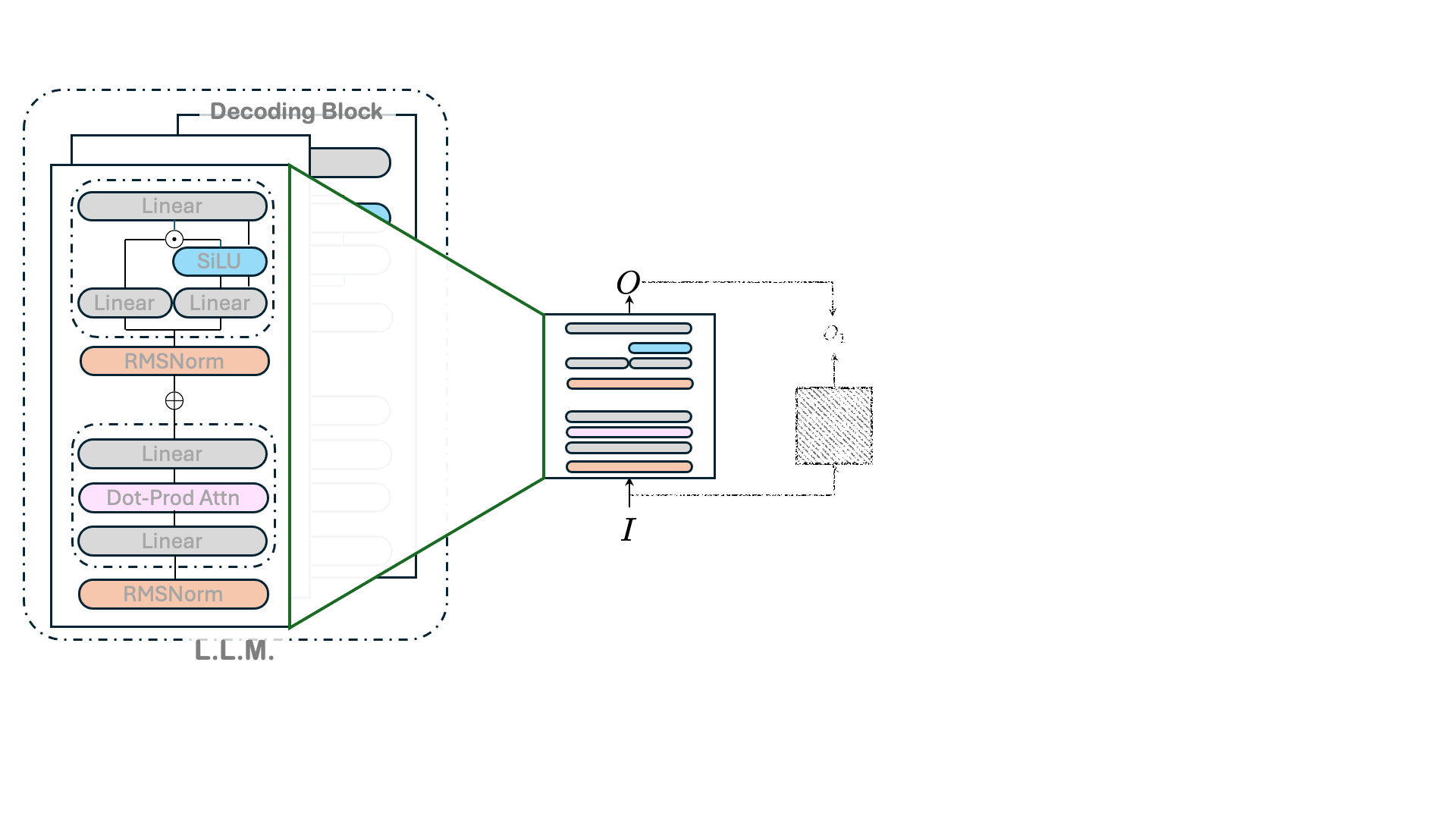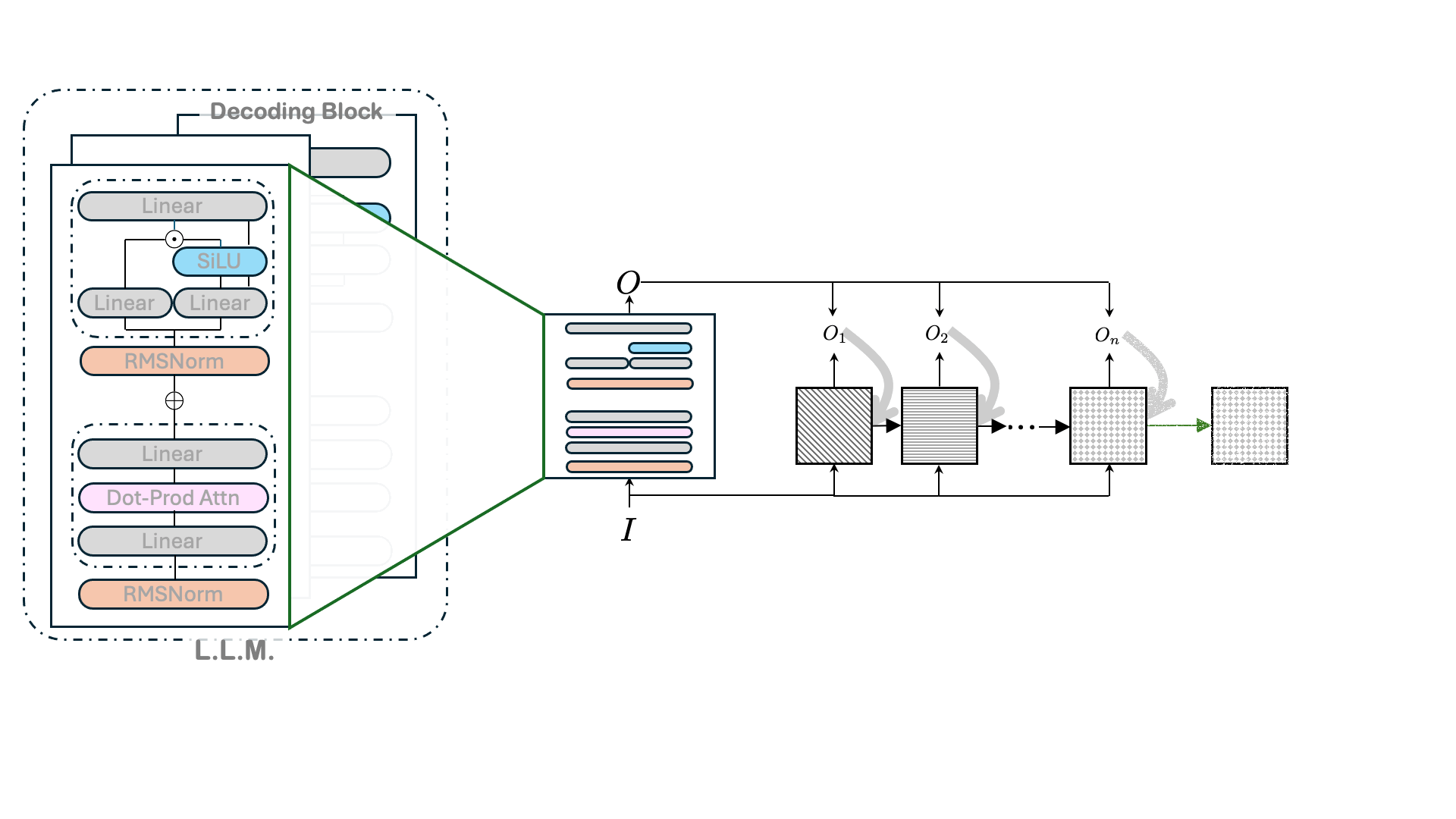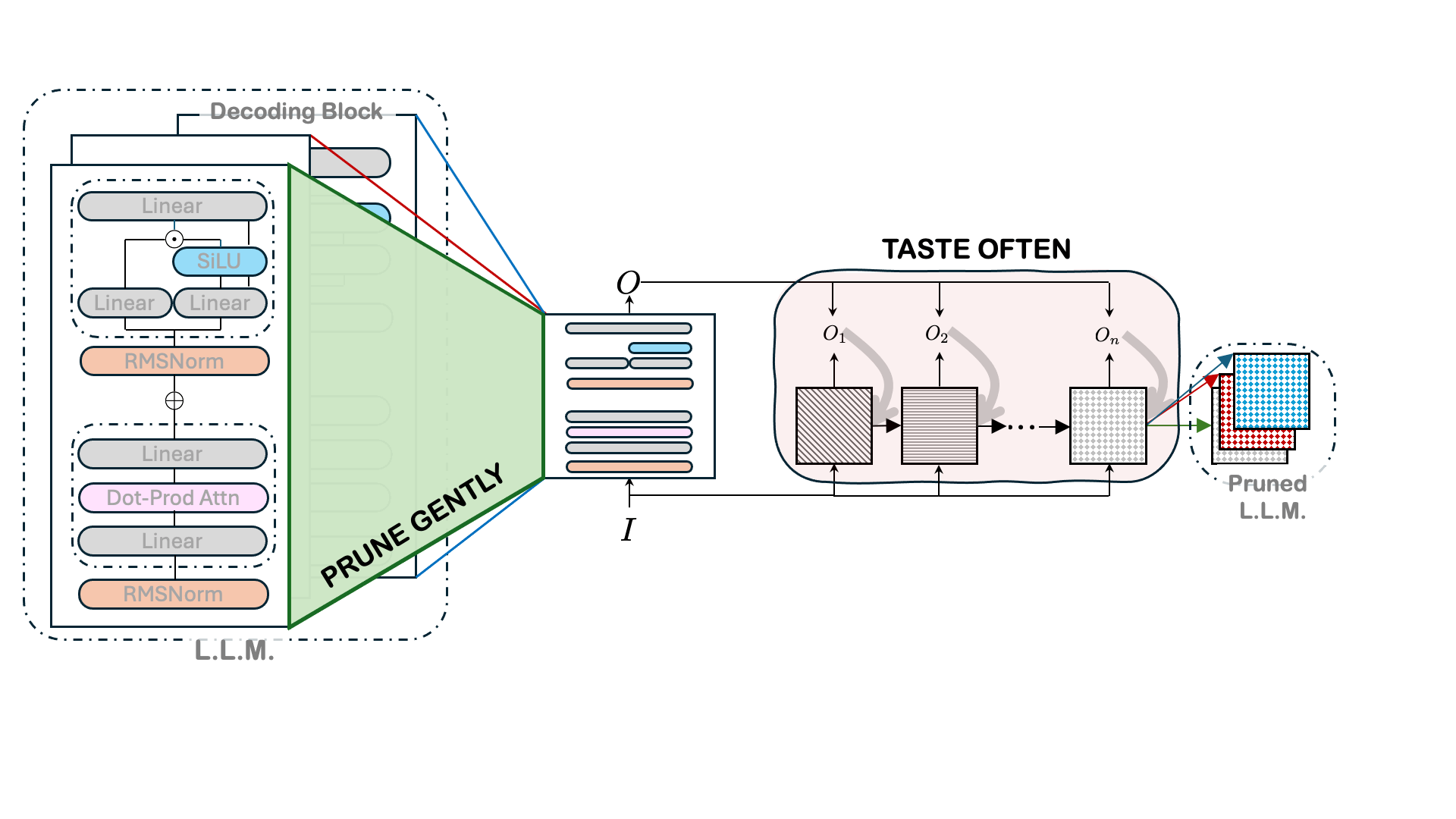
The overhead cost of performing universal fault-tolerant quantum computation for large scale quantum algorithms is very high. Despite several attempts at alternative schemes, magic state distillation remains one of the most efficient schemes for simulating non-Clifford gates in a fault-tolerant way. However, since magic state distillation circuits are not fault-tolerant, all Clifford operations must be encoded in a large distance code in order to have comparable failure rates with the magic states being distilled. In this work, we introduce a new concept which we call redundant ancilla encoding. The latter combined with flag qubits allows for circuits to both measure stabilizer generators of some code, while also being able to measure global operators to fault-tolerantly prepare magic states, all using nearest neighbor interactions. In particular, we apply such schemes to a planar architecture of the triangular color code family. In addition to our scheme being suitable for experimental implementations, we show that for physical error rates near 10−4 and under a full circuit level noise model, our scheme can produce magic states using an order of magnitude fewer qubits compared to the most competitive magic state distillation schemes. Further, we can take advantage of the fault-tolerance of our circuits to produce magic states with very low logical failure rates using encoded Clifford gates with noise rates comparable to the magic states being injected. Consequently, we believe our scheme to be suitable for implementing fault-tolerant universal quantum computation with hardware currently under development.
Research areas